Air Column Resonance

Purpose

To create standing waves in an air column with variable length.

Objectives

To create standing wave patterns in an open-end tube and to determine their wavelength
To learn how to record and organize experimental data using spreadsheets

Equipment

Plastic cylindrical tube connected to water container
Tuning fork, mallet, a pencil

Theory

The velocity of a wave with frequency f and wavelength $\lambda$ is given by

$v = \lambda f\;\;\;\;(\text{Eq.1})$

For a sound wave in air, the velocity depends on the temperature as

$v = 331+0.6T\;\;\;\;(\text{Eq.2})$

For standing wave patterns to be occur in a tube with one open and one closed end, the length of the tube L and the wavelength $\lambda$ must satisfy the following condition

$L = m \left(\frac{\lambda}{4}\right) \;\text{where }\; m=1,3, 5,\ldots \;\;\;\;(\text{Eq.3})$

In the experiment, the length of the tube is determined by the water level, y. Thus, the wavelength of the waves producing the standing wave pattern will be :

$\lambda = \frac{4 y}{m}\;m=1,3,5,7,\ldots\;\;\;\;(\text{Eq.4})$

Pre-lab Activity

The water level in a vertical glass tube 1.00 m long can be adjusted to any position in the tube. A tuning fork vibrating at 1024 Hz is held just over the open top end of the tube, in order to set up a standing wave of sound in the air-filled portion of the tube. Considering room temperature (20^oC), at what positions of the water level will sound from the fork set up resonance in the air column inside the tube?

Experimental Setting

Here is step-by-step detailed instructions on how to set up the lab.

Procedure

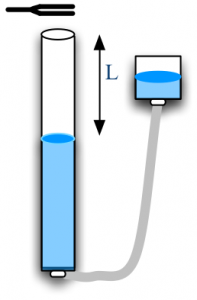Fill in the tube with water. Using the mallet, set the tuning fork to vibrate in its fundamental frequencies. Move the water container up and down and listen for the position where resonance occurs. Mark them in pencil on the outside surface of the tube.

Measure the room temperature and calculate the speed of sound in the room using Eq. 2.
Calculate the expected wavelength for the 512 Hz and 1024 Hz fork by using Eq.1

	T (^oC)	v (m/s)	wavelength (m)
512 Hz
1024 Hz

Things To Watch For!

Don’t spill water!

Use a pitcher and fill in the tube-container system with water. Be careful so that water does not spill. As you move the water container up and down, water level in the container and the tube will always be the same (water seeks its level). This is the way you can control the length of the air column in the plastic tube.

The standing waves are in the AIR-filled portion of the tube!

The standing waves are created in the air space between the opening of the tube and the water level. The resonance (standing waves) will occur only when the water is at such a level that the length of the air column L meets the conditions in Eq.3

Listen for the resonance of the fundamental frequency produced by the fork.

The sound becomes distinctively loud when the conditions of Eq.3 are met. There will be positions that you will hear fainter sound – those might be the positions for standing waves due to the higher harmonics. Generally, if you are unsure whether the sound is loud or not, it is most likely not the resonance you are looking for.

Data

Record your data in a data table.

	512 Hz	1024 Hz
y₁ in (m)
y₂ in (m)
y₃ in (m)
y₄ in (m)

Results

Using your measurements above, determine the wavelength for each fork by using Eq. 4. Each position y should yield the same value of wavelength – so use your measurements to determine those values, and average it. The average value is your best result for the wavelength.

Check your value for the wavelength against what it should be, and calculate the percent difference between the two.